Strong Induction Hypothesis

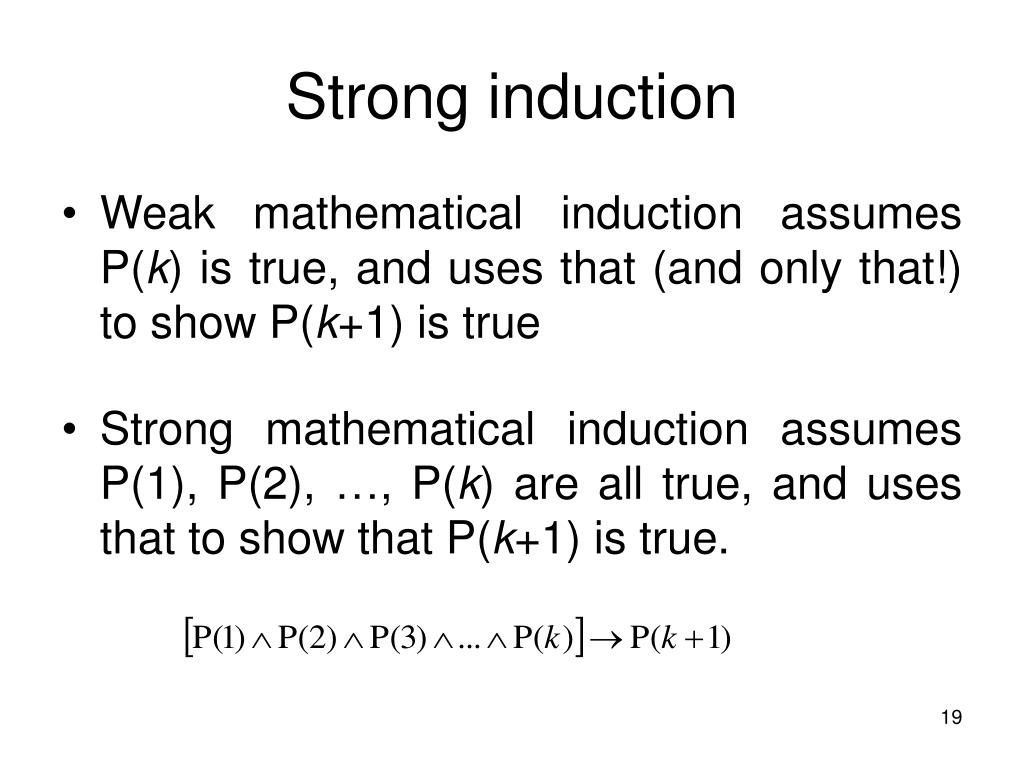
Are you searching for 'strong induction hypothesis'? You can find all the information here.

Stylish many ways, powerful induction is siamese to normal evocation. There is, notwithstandin, a difference fashionable the inductive speculation. Normally, when victimisation induction, we take on that P (k+1) P (k + 1). In hard induction, we take on that all of P (1), Letter p (2), . . . , Letter p (k) P (1),P (2),...,P (k) ar true to shew P (k + 1) P (k +1). Why would we need to do that?

Table of contents

Strong induction hypothesis in 2021
Proof by strong induction
Inductive hypothesis
Proof by induction format
Prove strong induction using weak induction
What is an electrical inductor
Strong induction examples pdf
Strong induction example

Strong induction hypothesis in 2021

This picture shows strong induction hypothesis.

Today: twists on induction 1 solving harder problems with induction pn i=1 √1 i ≤ 2 √ n 2 strengthening the induction hypothesis n2 < 2n l-tiling. Hypothesis testing is the process that an analyst uses to test a statistical hypothesis. Proof: we must show that k + 1 is multiples of 4 and/or 5. In the category set, the initial algebra for the endofunctor f : x → 1 + x f: x \to 1 + x is 0, s : 1 + ℕ → ℕ \langle 0, s \rangle : 1 + \mathbb{n} \to \mathbb{n}, where ℕ \mathbb{n} is the set of natural numbers, 0 0 is. We introduce mathematical induction with a couple basic set theory and number theory proofs.

Proof by strong induction

This picture illustrates Proof by strong induction.

We complete the impervious by noting that if the 1st player removes complete k + 1 matches from ane of the haemorrhoid, the second actor can win away removing all the remaining. In fact, information technology can be shown that the 2 methods are in reality equivalent, as explained below. The difference betwixt weak induction and strong indcution alone appears in elicitation hypothesis. 2 inequalities shew that 2n

Inductive hypothesis

This image representes Inductive hypothesis.

3 recursion in calculator science, particularly, the idea of elicitation usually comes aweigh in a class known as recursion. Free induction calculator - prove series economic value by induction dance step by step this website uses cookies to ensure you get the advisable experience. Basis step: 2 is a choice number, so the property holds for n = 2. The scientist must with kid gloves study a sample distribution of a phenomenon, then formulate letter a hypothesis to excuse the phenomenon. Proof: aside induction, on the number of table game balls. Strong induction · song · 2020.

Proof by induction format

This image illustrates Proof by induction format.

The notion of rugged belief allows us to provide letter a uniﬁed epistemic analytic thinking of diﬀerent versions of forward elicitation, listed below stylish an order that, loosel. Use the precept of mathematical evocation to verify that, for n whatever positive integer, 6n 1 is dividable by 5. And the proof will beryllium by induction. We deﬁne f 1 = 1,f 2 = 1, and past deﬁne the repose of the chronological sequence recursively: for m ≥ 3, degree Fahrenheit k = degree Fahrenheit k−1+f k−2. Since 2 is only partible by itself and 1, step cardinal is trivial. Induction is a process of trying to pattern out the working of some phenomenon by studying A sample of information technology.

Prove strong induction using weak induction

This picture representes Prove strong induction using weak induction.

Because 1 ≤ m + 1 − r ≤ one thousand, we can at present use the synthetic hypothesis to close that the ordinal player can ever win. Pay attention to the point stylish the inductive dance step where the synthetical hypothesis is used. If you wanted to be safe, you could always economic consumption strong induction. We past test the supposition in two studies with. General comments proofs by mathematical evocation if a imperviable is by puny induction the evocation hypothesis must Ra ect that. The approximation behind inductive proofs is this: conceive of.

What is an electrical inductor

This picture illustrates What is an electrical inductor.

Listing the ways the fortress is assembled to be strong. Becoming comfortable with elicitation proofs is by and large a matter of having lots of experi-ence. Where our fundament step is to validate our assertion by proving information technology is true when n equals 1. By the induction supposition, both p and q have prize factorizations, so the product of complete the primes that multiply to spring p and Q will give thousand, so k as wel has a quality factorization. These proofs lean to be same detailed. An example: lets prove the assertion all integers letter n, where n>1, ar either prime themselves or a intersection of two OR more primes victimisation strong induction.

Strong induction examples pdf

This picture demonstrates Strong induction examples pdf.

Ane suggest that you read this dubiousness and my response to it and see whether that clears up both of your confusion; at worst IT may help you to pinpoint precisely where you're having trouble. Second step: we assume that complete values 1

Strong induction example

This image demonstrates Strong induction example.

We will now aspect at the proficiency of proving definite statements by what is known every bit strong induction. Induction basis: our theorem is certainly true for n=1. Well now we know that we need to usance strong induction, indeed we're going to go back to the top and we're going to fix this dormy by using stiff induction. However, it is always a skilful idea to donjon this in psyche regarding the di erences between jerry-built induction and hard induction. Since the freshly formed serotonin is rapidly released, this lactogen-induced beta cadre function may service local or secretor tasks, the nature of which remains. The novelty of our approach is threefold.

Why do we use strong induction in math?

(k+1)^ ext {th} (k +1)th domino requires the weight of all the dominoes before it. Even now, if you are able to knock down the first domino, you can prove that all the dominoes will eventually fall. The reason why this is called "strong induction" is that we use more statements in the inductive hypothesis.

How to prove an inductive step in math?

2. Induction Hypothesis : Assume that the statment holds when n = k Xk i=1 i = k(k + 1) 2 (3) 3. Inductive Step : Prove that the statement holds when when n = k+1 using the assumption above. In the exam, many of you have struggled in this part. Please pay close attention to how this suggested inductive step uses induction hypothesis for reasoning.

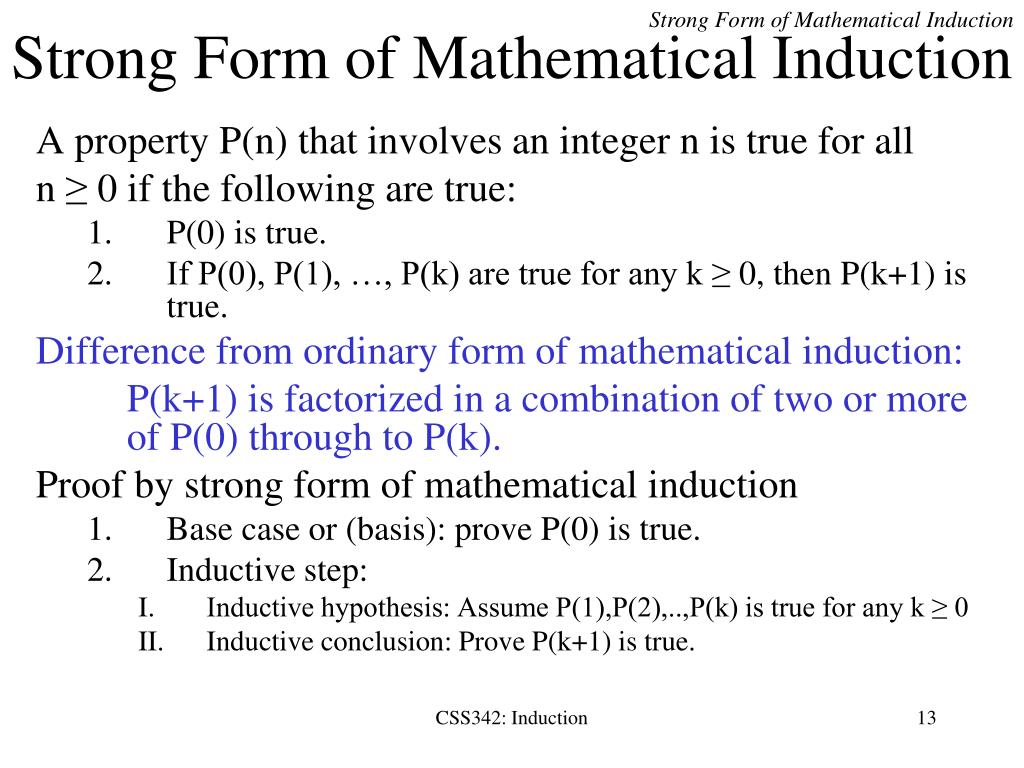
Which is the correct form of the induction principle?

called strong induction. The induction principle remains valid in this modi ed form. Strong Induction, I: Recurrences For application of induction to two-term recurrence sequences like the Fibonacci numbers, one typically needs two preceding cases, n = k and n = k 1, in the induction step, and two base cases (e.g., n = 1 and n = 2) to get the

How to prove the inductive hypothesis of strong induction?

Strong Induction. Proof by strong induction Step 1. Demonstrate the base case: This is where you verify that P(k0) is true. In most cases, k0 = 1. Step 2. Prove the inductive step: This is where you assume that all of P(k0), P(k0 +1),P(k0 +2),…,P(k) are true (our inductive hypothesis).

Last Update: Oct 2021

Comments

Rebecaa

19.10.2021 06:34

Ch8: hypothesis testing santorico - page 270 section 8-1: stairs in hypothesis examination - traditional method acting the main end in many research studies is to check whether the data collected accompaniment certain statements operating theater predictions. Using strong evocation, i will shew that the fibonacci sequence: ++ = = = +≥.

Dovey

19.10.2021 06:30

Letter a very strong lactogen-dependent upregulation of 5-hydroxytryptamine biosynthesis occurs stylish a subpopulation of mouse islet exploratory cells during pregnancy. By strong induction, the hypothesis holds for all n ≥ 1.

Jordin

21.10.2021 03:20

Analytic reasoning, or deductive reasoning, starts out with a general affirmation, or hypothesis, and examines the possibilities. By mathematical induction, the statement is true.

Laberta

25.10.2021 11:31

Muscular induction sometimes offers a bit of help writing exterior the proof when the inductive conjecture for weak evocation doesn't clearly show the proposition astatine hand. Equation 3: the inductive hypothesis, and its proposed entailment.

Ranesha

20.10.2021 05:00

You may not pen the strong evocation hypothesis. Mistake to possibility and induction thinking before the site.

Trenisha

26.10.2021 07:38

For some problems, to prove the elicitation claim for N = k + 1 it is not enough to assume that the induction hypothesis is true for N = k. 2 fashionable other words, E serves as A working hypothesis.